

Explanation of 9-Point Circle
by
Tizzy Shagginess
Write-up #4
What is the 9-Point Circle?
For any give triangle the midpoint of each side, the foot of each altitude, and the midpoint of the segment from each vertex to the orthocenter all lie on the same circle. This circle is called the 9 point circle.
Explanation of Proof of 9-Point Circle:
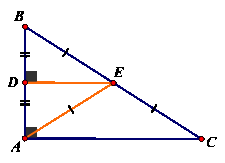
Before we explain the proof of the 9 point circle, we first must prove a theorem that we will use in the explanation. We will show that the midpoint of the hypotenuse is equidistant from all of the vertices of the tri
Consider right triangle ABC where angle BAC is the right angle. We want to show that the midpoint of the hypotenuse is equidistance from all three vertices of the triangle. Construct the midpoint of BC (the hypotenuse), call it E. By definition of a midpoint, segment BE is congruent to segment EC. Construct the midpoint of segment BA, call it D. By definition of a midpoint, segment AD is congruent to segment BD. Segment DE is parallel to segment AC so angle BDE and angle ADE are right angles. This makes triangle ADE and triangle BDE right triangles. These triangles have side DE in common and we know DA is congruent to DB. So by SAS we know triangle ADE and triangle BDE are congruent. This makes BE congruent to AE which makes segments BE, AE, and EC congruent. Thus the midpoint of the hypotenuse is equidistance from all three vertices. Triangle ABC is seen in the figure below.
The proof of the existence of the 9 point circle shows that the midpoints of the sides of the triangle, the foot of each altitude, and the midpoints of the segments that connect the vertices of the triangle to the orthocenter all lie on the same circle; this circle is called the 9 point circle. The proof proves this by showing that particular segments are parallel and therefore perpendicular to other segments. This allows for the construction of two rectangles whose corners are points on the 9 point circle. These rectangles can be inscribed in a circle; this circle is the nine point circle. This shows that 6 of the 9 points are on the same circle. The final three points can be proved to be on the circle using triangles and segments that create right angles and the fact that the midpoint of the hypotenuse of a right triangle is equidistant from the vertices of the triangle. The midpoint of the hypotenuse is the center of the 9 point circle which makes the segments from the midpoint of the hypotenuse to the vertex of the smaller triangles points on the circle.
Consider a triangle. The left most vertex of the triangle will be called the left, or bottom left, vertex, the vertex that is between the other two vertices will be called the top vertex, and the right most vertex will be called the right, or bottom right, vertex. Consider the segment created by the midpoint of the two legs of the triangle. This segment is parallel to the base of the triangle because if a line bisects one side of a triangle and bisects another side, then the line is parallel to the third side.
Consider the segment created by the left end of the base of the triangle and orthocenter and the segment created by the right end of the base of the triangle and the orthocenter. The midpoint of each of these two segments creates a segment that is parallel to the base of the triangle. Thus, this segment is parallel to the segment created by the midpoints of the legs of the triangle. These parallel segments are in orange in the figure below and the orthocenter is labeled O.

The bottom left vertex of the original triangle, the top vertex of the original triangle, and the orthocenter of the original triangle form a triangle. The side from the top vertex of the triangle to the orthocenter is part of an altitude of the original triangle because the orthocenter is the intersection of the altitudes of the original triangle. Construct the midpoint of the other two sides of the triangle and connect the midpoints to create a segment. Using the same logic as before, this segment is parallel to the side that is part of the altitude of the original triangle.
The bottom right vertex of the original triangle, the top vertex of the original triangle, and the orthocenter of the original triangle also form a triangle. Using the same process as before, the segment created by the midpoint of two of the sides is also parallel to the side that is part of the altitude of the original triangle. Therefore both segments created using the midpoints of the smaller triangles are parallel to each other.
The segment created by the midpoints of the sides of the smaller triangle is also parallel to segment that is the altitude of the original top vertex. These parallel segments are shown in orange in the figure below.
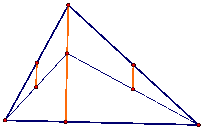
Consider the quadrilateral that is made up of the two pairs of parallel segments we have previously mentioned. The first set of segments is perpendicular to the altitude from the top vertex and the second set of segments is parallel to the same altitude so the sets of segments must be perpendicular to each other. Thus this quadrilateral forms a rectangle.
The opposite corners of the rectangle sum to 180 degrees therefore the rectangle can be inscribed in a circle. Three points define a circle so we must justify that the fourth vertex is on the same circle as the circle defined by the other three points. The fourth vertex must be on the same circle defined by the other three points because the center of the circle is also the center of the rectangle inscribed in it. The segments from center of the rectangle to the three vertices that define the circle are all radii of the circle. Because the figure is a rectangle, the fourth point is same distance from the center as the other vertices. But the center of the rectangle is also the center of the circle which makes the segment from the center to the fourth point a radius. Thus the fourth point is also on the circle that is defined by the other three points of the rectangle.
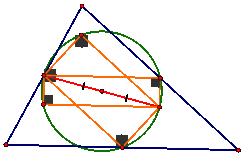
These first four points determine the circle that is the 9 point circle. This rectangle is seen in orange and the circle the points lie on is in green in the figure below.

Another quadrilateral is created by the midpoint of the base of the original triangle, the midpoint of the left leg of the original triangle, the midpoint of the segment connecting the bottom right vertex to the orthocenter, and the midpoint of the segment connecting the top vertex to the orthocenter. Using the same logic that was used with the previous rectangle, this new quadrilateral can be inscribed in a circle. The new rectangle is in orange and the circle is in green in the figure below.

Two of the corners of this rectangle are also corners of the first rectangle so the circle that inscribes the new rectangle must be the same circle that inscribes the first rectangle. So now we have 6 of the 9 points on the 9 point circle. If we were to repeat this process a third time we would not get another rectangle so we must use a different technique.
The final three points we need to show are on the circle are the points where the altitude of each vertex intersects the opposite side. The midpoint of the left leg of the triangle and midpoint of the segment connecting the bottom right vertex to the orthocenter are vertices of both of the rectangles. Therefore the segment connecting these two points is a diagonal for both of the constructed rectangles. These points are both on the circle so we can say that this segment is a diameter of the circle with the midpoint of the segment being the center of the circle. This diameter is in red in the figure below.
The segment from the midpoint of the segment connecting the top vertex to the orthocenter and midpoint of the base of the triangle is also a diameter of the circle because the segments connecting these points goes through the center of the circle. The end points of this diameter and the point where the altitude of the top vertex intersects the base of the triangle can create a triangle. By definition of an altitude the angle created by the point where the altitude intersects the base of the triangle forms a right angle and thus these three points form a right triangle. The midpoint of the hypotenuse is the center of the circle and the midpoint of a hypotenuse is equidistant from all of the vertices of the triangle. (The proof that the midpoint of the hypotenuse is equidistant from the vertices was given before explanation) This right triangle is shown in red in the figure below and the two of the radii are shown by the dotted purple line.
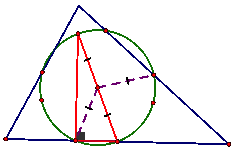
This shows that 1 of the 3 three points we still needed to prove is on the circle is indeed on the 9 point circle. This same process can be applied to the other altitudes of the vertices to show that the remaining two points are on the 9 point circle. Therefore we have shown that all of the 9 points exist on the 9 point circle, as shown in the figure below.
